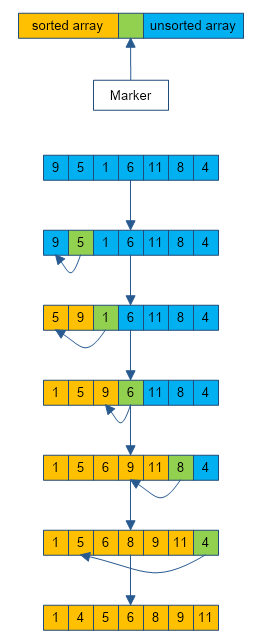
Sort a given unsorted array using the insertion sort algorithm.
Input: {9,5,1,6,11,8,4}
Output: {1,4,5,6,8,9,11}
Table of Contents
Theory
- Insertion Sort sorts numbers in the same way as we humans sort a set of numbered objects (ex cards)
- A number is taken from an unsorted array (right subarray) to a position in the sorted array (left subarray) such that the left subarray remains sorted.
- It is an incremental approach-based method.
Insertion Sort Algorithm
- Select/Mark the first element in an unsorted array, move it to the correct position in the sorted array.
- Advance the marker to the next element in an unsorted array.
C++ Program
#include <iostream>
using namespace std;
void insertSort(int arr[],int n)
{
int i,j,save;
for(int i=1;i<n;i++)
{
j = i-1;
save = arr[i];
// look for correct position of arr[i]
while(j>=0 && arr[j] > save)
{
// shifting array elements towards the right
arr[j+1] = arr[j];
j--;
}
// place arr[i] at the correct position
arr[j+1] = save;
}
}
int main()
{
int arr[] = {9,5,1,6,11,8,4};
int n = sizeof(arr)/sizeof(arr[0]);
insertSort(arr,n);
for(int i=0;i<n;i++)
cout<<arr[i]<<" ";
return 0;
}Output
1 4 5 6 8 9 11
Java Program
class iSort
{
static void insertSort(int arr[])
{
int n = arr.length;
int i,j,save;
for(i=1;i<n;i++)
{
j = i-1;
save = arr[i];
// look for correct position of arr[i]
while(j >= 0 && arr[j] > save)
{
// shifting array elements towards the right
arr[j+1] = arr[j];
j--;
}
// place arr[i] at the correct position
arr[j+1] = save;
}
}
public static void main(String args[])
{
int arr[] = {9,5,1,6,11,8,4};
insertSort(arr);
for(int i=0;i<arr.length;i++)
System.out.print(arr[i] +" ");
}
}Output
1 4 5 6 8 9 11
Complexity Analysis
- Time Complexity: T(n) = O(n2)
- It takes O(n2) time when an array is reversely sorted and O(n) time when the array is sorted.
- Space Complexity: A(n) = O(1)
Supplementary Information
- Insertion Sort is an in-place sorting algorithm.
- It is a stable nature.
- Insertion Sort is useful when the input array is almost sorted, only a few elements are misplaced incomplete big array.
- It is also useful when the array to be sorted is smaller in size.