Given a list of words, find the longest common prefix of all the words by word by word matching.
Table of Contents
Examples
Input:
list[] = {“apple”, “ape”, “april”}
Output:
ap
Input:
list[] = {“star”, “stole”, “steam”, “start”}
Output:
st
Algorithm for Longest Common Prefix
The longest common prefix of two words is found as,
Let W1 be the first word and W2 be the second word,
- Initialize a string variable commonPrefix as “”(empty string).
- Start traversing in W1 and W2 simultaneously, till we reach the end of any one of the words.
- Match the characters of W1 and W2, if these are equal append it to commonPrefix and advance in both the words.
- Else return commonPrefix
The above algorithm can be extended to find the longest common prefix of list of N words as,
- Initialize a string variable commonPrefix as first word in the list.
- Traverse in the list starting from index 1(o based indexing).
- Update commonPrefix as a common prefix of commonPrefix and current word in the list.
- At the end of the traversal return commonPrefix.
Explanation for Longest Common Prefix
Consider an example,
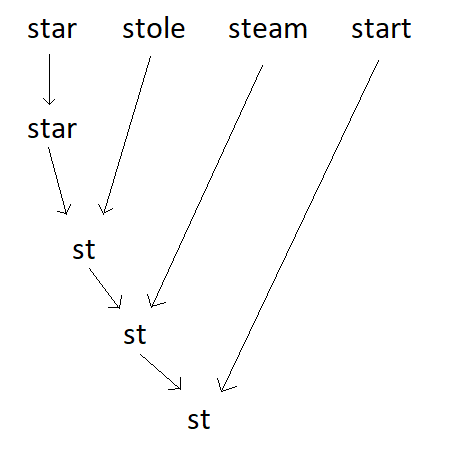
list[] = {“star”, “stole”, “steam”, “start”}
Step 1
commonPrefix = “star”
Step 2
Repeat step 3 and 4 while traversing the list from index 1 to end of the list.
Step 3 and 4
Iteration 1
commonPrefix= common prefix of (commonPrefix, “stole”)
commonPrefix = “st”
Iteration 2
commonPrefix= common prefix of (commonPrefix, “steam”)
commonPrefix = “st”
Iteration 3
commonPrefix = common prefix of (commonPrefix, “start”)
commonPrefix = “st”
We have traversed the list and the common prefix of all the words in the list is “st”. See the image below for clarification.
JAVA Code
public class LongestCommonPrefixUsingWordByWordMatching {
// Function to find common prefix of two words
private static String prefix(String W1, String W2) {
// Initialize prefix as empty string
String prefix = "";
int n1 = W1.length();
int n2 = W2.length();
int i = 0, j = 0;
// Traverse in W1 and W2 simultaneously
while (i < n1 && j < n2) {
// If characters of W1 and W2 are equal, append it to answer else return prefix
if (W1.charAt(i) == W2.charAt(j)) {
prefix += W1.charAt(i);
i++;
j++;
} else {
return prefix;
}
}
// All words matched, return prefix
return prefix;
}
private static String longestCommonPrefix(String[] list) {
// Initialize longest common prefix as first word of list
String lcp = list[0];
// Traverse the list from index 1 (0 based indexing)
for (int i = 1; i < list.length; i++) {
// Update lcp as prefix of lcp and current word
lcp = prefix(list[i], lcp);
}
// return lcp
return lcp;
}
public static void main(String[] args) {
// Example 1
String list1[] = new String[] {"apple", "ape", "april"};
System.out.println(longestCommonPrefix(list1));
// Example 2
String list2[] = new String[] {"star", "stole", "steam", "start"};
System.out.println(longestCommonPrefix(list2));
}
}C++ Code for Longest Common Prefix
#include <bits/stdc++.h>
using namespace std;
// Function to find common prefix of two words
string prefix(string W1, string W2) {
// Initialize prefix as empty string
string prefix = "";
int n1 = W1.size();
int n2 = W2.size();
int i = 0, j = 0;
// Traverse in W1 and W2 simultaneously
while (i < n1 && j < n2) {
// If characters of W1 and W2 are equal, append it to answer else return prefix
if (W1[i] == W2[j]) {
prefix += W1[i];
i++;
j++;
} else {
return prefix;
}
}
// All words matched, return prefix
return prefix;
}
string commonPrefix(vector<std::string> &list) {
// Initialize longest common prefix as first word of list
string lps = list.front();
// Traverse the list from index 1 (0 based indexing)
for (auto itr = list.begin() + 1; itr != list.end(); itr++) {
// Update lcp as prefix of lcp and current word
lps = prefix(lps, *itr);
}
// return lcp
return lps;
}
int main() {
// Example 1
vector<std::string> list1;
list1.push_back("apple");
list1.push_back("ape");
list1.push_back("april");
cout<<commonPrefix(list1)<<endl;
// Example 2
vector<std::string> list2;
list2.push_back("star");
list2.push_back("stole");
list2.push_back("steam");
list2.push_back("start");
cout<<commonPrefix(list2)<<endl;
return 0;
}ap st
Complexity Analysis for Longest Common Prefix
Time Complexity = O(N * maximum length of a word in the list)
Space Complexity = O(1)
where N is the total number of words in the list.