Quick Sort

Quick Sort is a sorting algorithm. Given an unsorted array sort it using quick sort algorithm.

Example

Input: {8, 9, 5, 2, 3, 1, 4}

Output: {1, 2, 3, 4, 5, 8, 9}

Theory

1. It’s a Divide and Conquer sorting Algorithm.
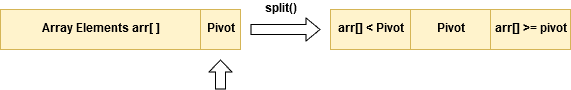
2. It picks a pivot element in the array, splits the array into two parts. One part consists of array elements having a value less than the pivot, another part contains array elements greater than the pivot. It then places the pivot in between these parts and recursively sorts left and right subarrays.
3. Pivot element can be selected in the following ways :
   1. first array element
   2. last array element (implemented here)
   3. median array element
   4. Any Array element.
4. After selecting pivot we apply the split() function to split the rest of the array around the pivot.
   1. split() places array elements less than pivot towards left of the pivot
   2. split() places array elements greater than pivot towards the right of the pivot
   3. the left and right subarrays (of the pivot) may or may not be sorted
5. we recursively sort left and right subarrays to obtain completely sorted array.

Quick Sort Algorithm

we mainly apply two functions

1. split() – select pivot and split the array around the pivot, we select the last element as pivot.
2. quickSortServe() – recursively sort left and right subarrays.

C++ Code for Quick Sort

#include <iostream>
#include <bits/stdc++.h>
using namespace std;

// funtion to split array around pivot
int split(int arr[],int lo,int hi)
{
    int i = lo-1;
    // select last element as pivot
    int pivot = hi;

    // All elements less than arr[pivot] are brought to left side
    // This splits array into two parts
    // array -> [left subarr] [pivot] [right subarr]
    for(int j=lo;j<pivot;j++)
    {
        if(arr[j] < arr[pivot])
        {
            i++;
            swap(arr[i],arr[j]);
        }
    }

    // Bring pivot element to it's correct postion in sorted array
    // by swapping smallest element of right subarray with pivot
    swap(arr[i+1],arr[pivot]);

    return i+1;
}

// Service function to recursively perfrom split()
// for left and right subarrays
void quickSortServe(int arr[],int lo,int hi)
{
    if(lo < hi)
    {
        int pivot = split(arr,lo,hi);
        
        // recursively perfrom split() for right and left subarrays
        quickSortServe(arr,lo,pivot-1);
        quickSortServe(arr,pivot+1,hi);
    }
}

// Function to implement quick sort algorithm
void quickSort(int arr[],int size)
{
    quickSortServe(arr,0,size-1);
}

int main()
{
    
    int arr[] = {8,9,5,2,3,1,4};
    int n = sizeof(arr)/sizeof(arr[0]);

    quickSort(arr,n);

    for(int i=0;i<n;i++)
    cout<<arr[i]<<" ";
    
    return 0;
}

Output

1 2 3 4 5 8 9

Java Code for Quick Sort

import java.io.*;

class QSort
{
    int split(int arr[],int lo,int hi)
    {
        int i = lo-1;
        // select last element as pivot
        int pivot = hi;

        // All elements less than arr[pivot] are brought to left side
        // This splits array into two parts
        // array -> [left subarr] [pivot] [right subarr]
        for(int j=lo;j<pivot;j++)
        {
            if(arr[j] < arr[pivot])
            {
                i++;
                int temp = arr[j];
                arr[j] = arr[pivot];
                arr[pivot] = temp;
            }
        }

        // Bring pivot element to it's correct postion in sorted array
        // by swapping smallest element of right subarray with pivot

        int temp = arr[i+1];
        arr[i+1] = arr[pivot];
        arr[pivot] = temp;

        return i+1;
    }
    
    // Service function to recursively perfrom split()
    // for left and right subarrays
    void quickSortServe(int arr[],int lo,int hi)
    {
        if(lo < hi)
        {
            int pivot = split(arr,lo,hi);
            
            // recursively perfrom split() for right and left subarrays
            quickSortServe(arr,lo,pivot-1);
            quickSortServe(arr,pivot+1,hi);
        }
    }
    
    // Function to implement quick sort algorithm
    void quickSort(int arr[],int size)
    {
        quickSortServe(arr,0,size-1);
    }
    // main function
    public static void main(String args[])
    {
        int arr[] = {8,9,5,2,3,1,4};
        int n = arr.length;

        QSort obj = new QSort();
        obj.quickSort(arr,n);

        for(int i=0;i<n;i++)
        {
            System.out.print(arr[i]+" ");
        }
    }
}

Output

1 2 3 4 5 8 9

Complexity Analysis of Quick Sort

Time Complexity

1. 1. Best case: O(nlogn)
   2. Worst case: O(n²)
   3. Average case: O(nlogn)

Supplementary Information

1. Quicksort is not a stable sorting algorithm.
2. Quicksort is an in-place sorting algorithm – doesn’t require auxiliary space.
3. In Practice, quicksort is faster than Merge and Heap sort in cases where data is small and/or stored in external storage space.
4. Quicksort performs better than Merge sort in case of arrays and requires no extra space for sorting purposes.
5. It is also a cache-friendly sorting algorithm as it has a good locality of reference when used for arrays.
6. It is also tail recursive, therefore tail-call optimizations are done.
7. Merge Sort is preferred over Quick sort for sorting linked lists.

Reference Interview Questions